√完了しました! y=e^x slope field 171322-Y=e^x slope field
Calculus 1 The differential equation dy/dx equals x2/y2 I produces a slope field with horizontal tangents at y = 2 II produces a slope field with vertical tangents at y = 2 III produces a slope field with columns of parallel segments You can view more similar questions or ask a new question71 Slope Fields and Euler's Method Def An equation involving a derivative is called a differential equation The order of a differential equation is the order of the highest derivative involved in the equation Find all functions y that satisfy dy sec 2 52 xx dx Ans y = tan x x2 5x C General Solution Initial Value ProblemSketch slope field for y' = e^x y How do the solutions behave as x grows?
Slope Fields Teaching Calculus
Y=e^x slope field
Y=e^x slope field-Consider the differential equation given by dy/dx = xy/2 A Let y=f(x) be the particular solution to the given differential equation with the initial condition Based on the slope field, how does the value of f(02) compare to calculusSketch slope field for y' = x^2 Sketch slope field




First Order Differential Equations Pdf Free Download
A) y = 1− x2 d) y = cosx b) y = 1−x2 e) y = 1 1x2 c) y = ex 2Slope field of y ′ = x y with a graph of solutions satisfying , y ( 0) = 02, , y ( 0) = 0, and y ( 0) = − 02 🔗 By looking at the slope field we get a lot of information about the behavior of solutions without having to solve the equationFree slope calculator find the slope of a line given two points, a function or the intercept stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy
Each segment has the same slope as the solution curve through (x, y) and so is tangent to the curve there The resulting picture is called a slope field(or direction field) and gives a visualization of the general shape of the solution curves Figure 162a shows a slope field, with a particular solution sketched into it in Figure 162b I got y = e^(x^3/3) 2 Calculus!!The exact solution to the IVP, which is found with separation of variables, is y = e x 2 / 2 so the exact value of y(1) is e 1/2 ≈ The smaller value of h, therefore, yields a better approximation We graph the approximations obtained with h = 01 and h = 005 as well as the graph of y = e x 2 / 2 in Figure 226
Given, as given equation is not complete soo, it may be like (dy/dx) = y^2 /x or dy/dx y^2 /x = 0 1 if we take first condition , ie dy/dx = y^2 /x dy/y^2 = dx/ x take integration on both side integral{dy/y^2} = integral {dx/x} 1/y = log x logSee the answer See the answer See the answer done loading a) Sketch the slope field for y'=y/x along with several solution curves Expert Answer Who are the experts?(A) y=x2 (B) y=ex y=e−x (D) y=cosx (E) y=lnx 2 At the right is a slope field



Www Swl K12 Oh Us Downloads Slope fields Pdf




Slope Fields Teaching Calculus
The solution to the ODE y ′ = y − x is y = c e x x 1, however, this is inconsistent with the slope field that I have created Consider the curve y − x = C The slopes of the tangent lines of a solution along this curve are all y ′ = ( x C) ′ = 1, and each point in the x, y − plane is on a line of the form y = x C for some C In our case f (x,y) = x 4 (3 −y) The "computational way" to draw the slope field is to do what a simple common plotting software would do make the infinitesimal quantities not infinitesimal and turn the equation to Δy Δx = x 4 (3 − y) Then substitute some values and plot the results of the computations This discretization processCan you guess a particular solution by looking at the slope field?
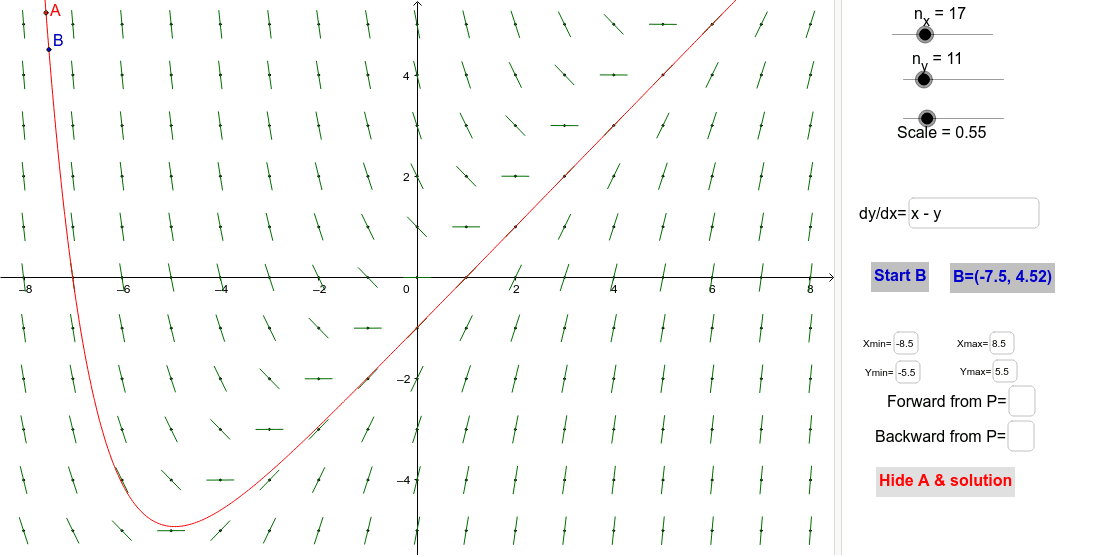



Slope Fields Geogebra




Slope Field For Y E X Novocom Top
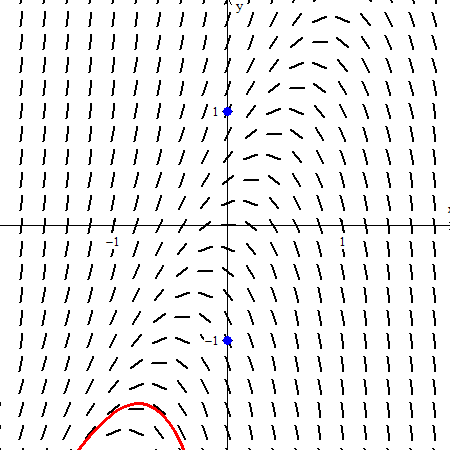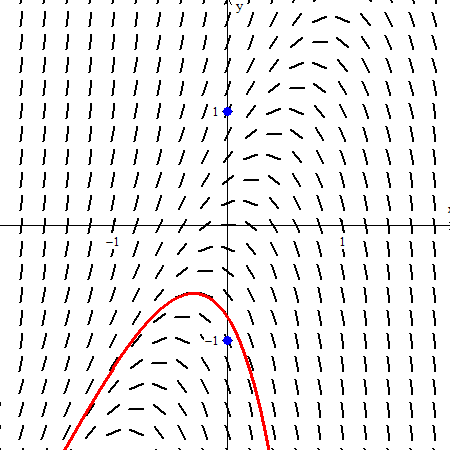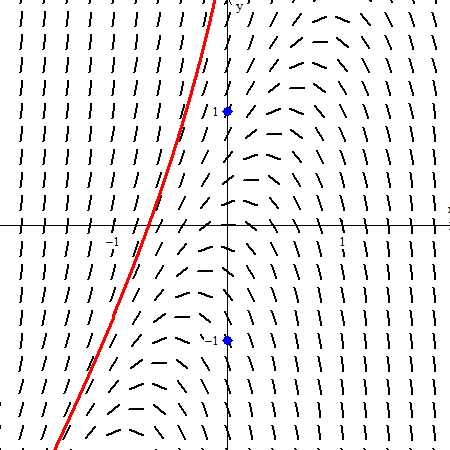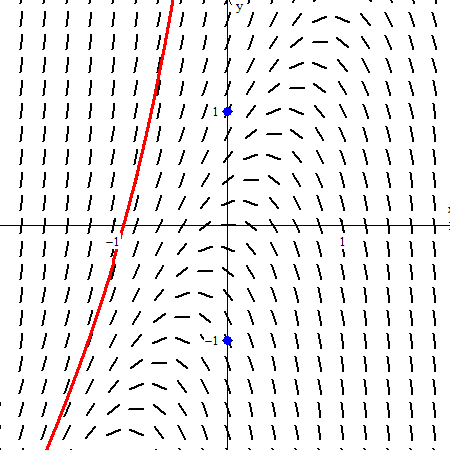
(a) Find a slope field whose integral curve through (0,0) satisfies x e^{y}y e^{x}=0 by differentiating this equation implicitly (b) Prove that if y(x) is an Join our free STEM summer bootcamps taught by expertsThe slope field of a de is based on the fact that the de can be interpreted as a statement about the slopes of its solution curves EXAMPLE 1 The de tells us that at any point (x, y) on a solution curve the slope of the curve is equal to its ycoordinate Since the de says that y is a function whose derivative is also y, we know that y = e xThe slope field for a certain differential equation shown below Which of the following could be a solution to the differential equation with the initial condition y(0) = 1?
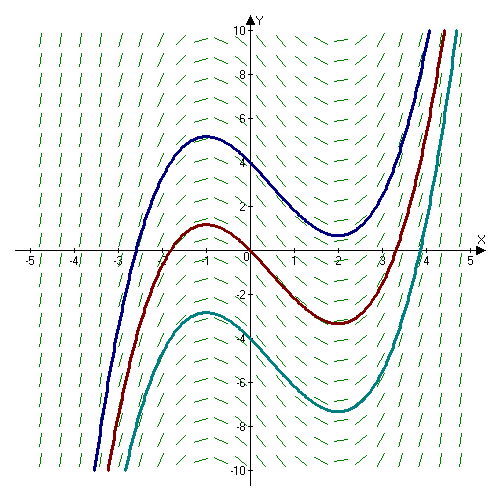



Slope Field Wikipedia



2
A direction field (slope field) is a mathematical object used to graphically represent solutions to a firstorder differential equation At each point in a direction field, a line segment appears whose slope is equal to the slope of a solution to the differential equation passing through that pointD y d x = x − y x 2 y y ( 0) = 1 Note that solving this exact equation will lead to an implicit formula for y which will have two solutions for x = 2 However, the initial value problem has a unique solution and so just one of the two solutions produced from the implicit formula will be the actual value of yDirection Field The slope field of a differential equation {eq}\displaystyle y'(x) = f(x,y) {/eq} shows the direction of the solution {eq}\displaystyle y(x) {/eq}
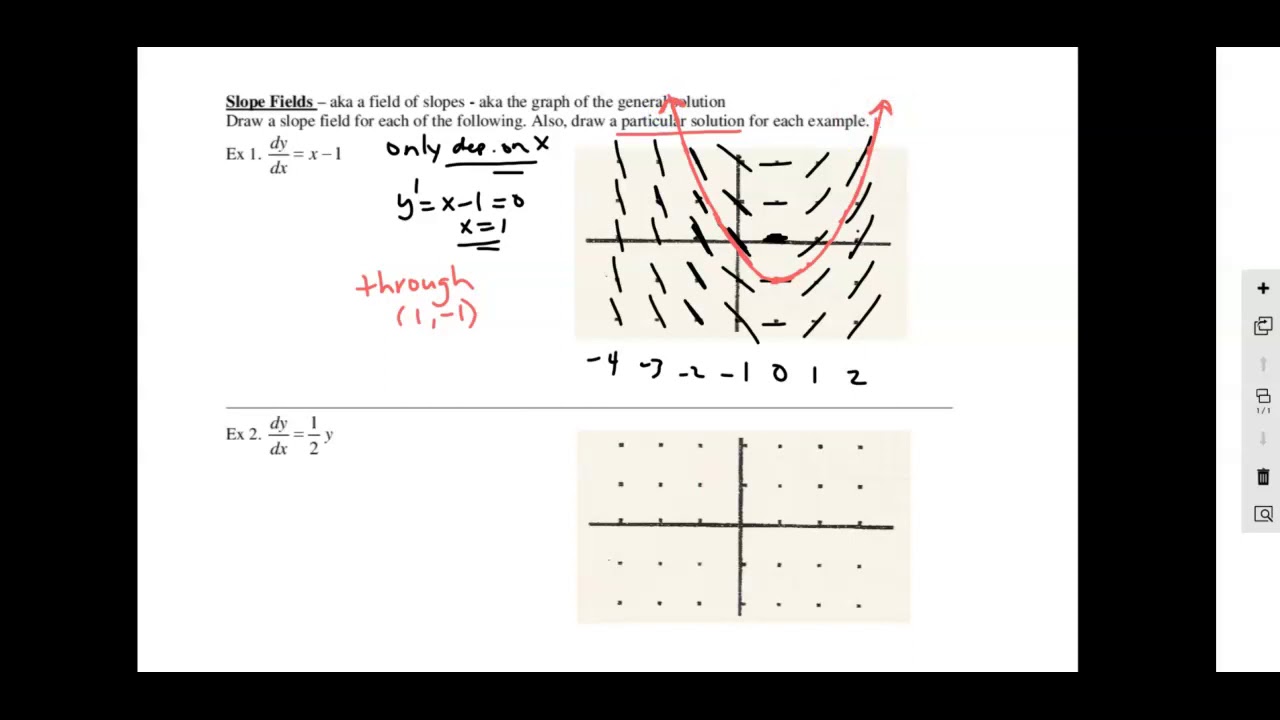



Calculus Slope Fields Part 1 Youtube



Http Www Manasquanschools Org Cms Lib6 Nj Centricity Domain 117 5 1 notes ab Pdf
Here's a slope field for the DE with superimposed plots of for (blue), (red), and (cyan) Here are the details for simplifying WolframAlpha's solution to get Mathematica's solution We use the recurrence relation With , we have y E) x y F) x y G) x y H) x y 7 y 1 dy dx 8 y x dy dx 9 yx2 dy dx 10 1 3 6 y x dy dx 11 2 1 y x dy dx 12 y sin( )x dy dx 13 y cos( )x dy dx 14 y ln x dy dx Show work on a separate piece of paper Integrate the 3 equations that it is possible to integrate Match the slope field with their differential equations A)162 Slope Fields X Y 0 0 1 1 2 2 3 3 4 4 Figure A slope field for y′(x) = 1 16 x 9− y2, along with four curves sketched "parallel" to the field 5



Slope Fields




How To Draw Slope Fields With All The Possible Solution Curves In Latex Tex Latex Stack Exchange



コメント
コメントを投稿